Mitch Richling: Tinkerbell Fractal
| Author: | Mitch Richling |
| Updated: | 2024-09-07 |
Table of Contents
1. Introduction
The Tinkerbell Attractor is a discrete-time dynamical system defined by the following relationship:
2. Algorithms
Just as with the Peter de Jong attractors we look at the probability density function,
#include "ramCanvas.hpp" std::vector<std::array<double, 11>> params { /* a, b, c, d, x0, y0, N, x-min, x-max, y-min, y-max */ { 0.900000, -0.220000, 2.0000000, 0.500000, 0.1, 0.1, 1e7, -1.410000, 0.370000, -1.380000, -0.350000 }, // 0 { 0.200000, 0.150000, 2.0000000, 0.650000, 0.1, 0.1, 1e7, -1.460000, 0.950000, -1.240000, -0.150000 }, // 1 { 0.900000, -0.601300, 2.0000000, 0.500000, 0.1, 0.1, 1e7, -1.240000, 0.470000, -1.560000, 0.550000 }, // 2 { -0.783671, -0.450251, 0.0756921, 1.908150, 0.1, 0.1, 1e8, -0.784949, 0.190357, 0.100000, 1.012020 }, // 3 { -0.667316, 0.789788, 1.1057300, 0.268078, 0.1, 0.1, 1e8, -0.903469, 1.087990, -0.708937, 0.319083 }, // 4 { -1.016430, -1.458560, 1.3230100, 0.604218, 0.1, 0.1, 1e8, -0.399636, 0.403479, -0.562466, 0.225223 }, // 5 { -0.254258, 0.925243, 0.7704410, -0.416270, 0.1, 0.1, 1e8, -0.888853, 0.939351, -0.734047, 0.346012 }, // 6 { -1.548590, -0.249840, -0.2864630, 1.508330, 0.1, 0.1, 1e8, -0.849159, 1.357350, -0.054140, 1.033830 }, // 7 { -0.769882, -0.743097, -1.0527500, 0.216079, 0.1, 0.1, 1e8, -0.864322, 1.141670, -0.394488, 0.686611 }, // 8 { -0.305249, -0.507228, -1.5821000, 0.919462, 0.1, 0.1, 1e8, -1.234830, 0.745791, 0.300000, 0.881405 }, // 9 { -1.754900, -0.904038, 0.9181340, 1.379920, 0.1, 0.1, 1e8, -0.821663, 1.448790, -2.112250, -0.100000 }, // 10 { -1.659840, -1.286440, 0.7102750, 1.097500, 0.1, 0.1, 1e8, -0.412856, 0.803154, -1.709030, -0.800778 }, // 11 { -1.733520, -0.647135, 0.5964760, 0.988405, 0.1, 0.1, 1e8, -0.700000, 1.800000, -0.312000, -0.273000 }, // 12 }; int main(void) { std::chrono::time_point<std::chrono::system_clock> startTime = std::chrono::system_clock::now(); const int BSIZ = 480*8; mjr::ramCanvas1c16b::colorType aColor; aColor.setChans(1); for(decltype(params.size()) j=0; j<params.size(); ++j) { mjr::ramCanvas1c16b theRamCanvas(BSIZ, BSIZ, params[j][7], params[j][8], params[j][9], params[j][10]); double a = params[j][0]; double b = params[j][1]; double c = params[j][2]; double d = params[j][3]; /* Draw the Attractor on a 16-bit, greyscale canvas such that the level is the hit count for that pixel. Thus we are using an "image" as a way to store field data instead of color information. */ double x = params[j][4]; double y = params[j][5]; uint64_t maxII = 0; uint64_t inCnt = 0; uint64_t maxItr = static_cast<uint64_t>(params[j][6]); uint64_t iPrt = maxItr / 5; uint64_t itr; for(itr=1;itr<maxItr;itr++) { double xNew = x*x-y*y+a*x+b*y; double yNew = 2*x*y+c*x+d*y; if ( !theRamCanvas.isCliped(x, y)) { inCnt++; theRamCanvas.drawPoint(x, y, theRamCanvas.getPxColor(x, y).tfrmAdd(aColor)); if(theRamCanvas.getPxColor(x, y).getC0() > maxII) { maxII = theRamCanvas.getPxColor(x, y).getC0(); if(maxII > 16384) { // 1/4 of max possible intensity std::cout << "ITER(" << j << "): " << itr << " MAXS: " << maxII << " EXIT: Maximum intensity reached" << std::endl; break; } } } if((itr % iPrt) == 0) std::cout << "ITER(" << j << "): " << itr << " MAXS: " << maxII << " INC: " << inCnt << std::endl; x=xNew; y=yNew; } std::cout << "ITER(" << j << "): " << itr << " MAXS: " << maxII << " INC: " << inCnt << std::endl; // Log image transform theRamCanvas.applyHomoPixTfrm(&mjr::ramCanvas1c16b::colorType::tfrmLn1); maxII = static_cast<uint64_t>(std::log(static_cast<double>(maxII))); /* Create a new image based on csCCfractal0RYBCW -- this one is 24-bit RGB color. */ mjr::ramCanvas3c8b anotherRamCanvas(BSIZ, BSIZ); typedef mjr::ramCanvas3c8b::colorType::csCCfractal0RYBCW cs_t; for(int yi=0;yi<theRamCanvas.getNumPixY();yi++) for(int xi=0;xi<theRamCanvas.getNumPixX();xi++) anotherRamCanvas.drawPoint(xi, yi, cs_t::c(theRamCanvas.getPxColor(xi, yi).getC0() / static_cast<mjr::ramCanvas3c8b::csFltType>(maxII))); anotherRamCanvas.writeTIFFfile("tinkerbell_" + mjr::fmtInt(j, 2, '0') + ".tiff"); } std::chrono::duration<double> runTime = std::chrono::system_clock::now() - startTime; std::cout << "Total Runtime " << runTime.count() << " sec" << std::endl; return 0; }
Source Link: tinkerbell.cpp
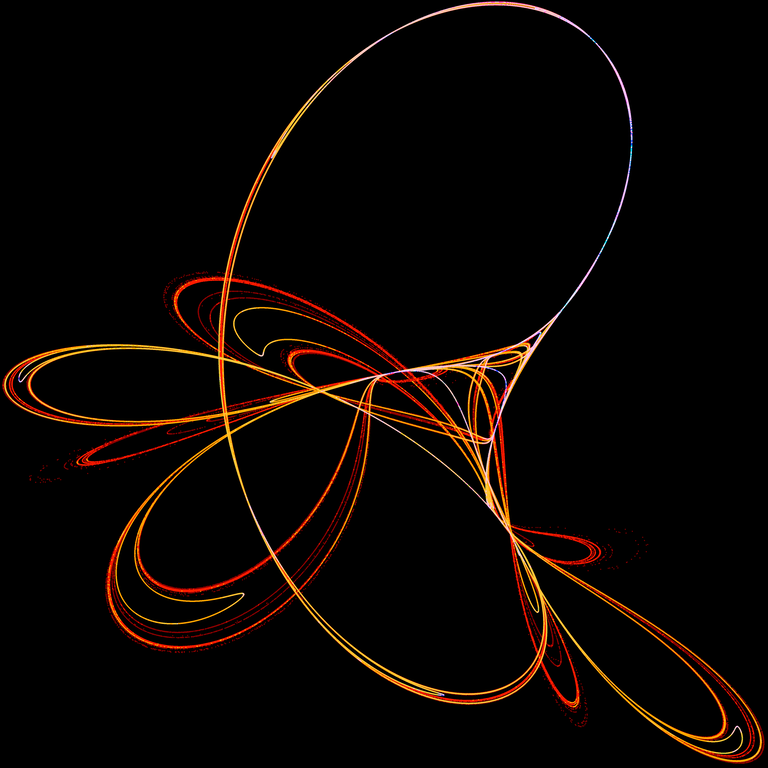
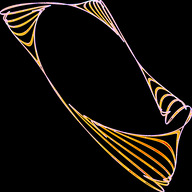
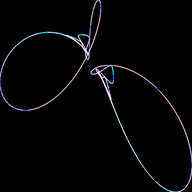
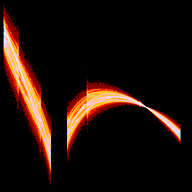
The above program will generate several images includeing some that have the "Classic Tinkerbell Atractor" shape: